Quantum computing promises ultra-powerful computational capability via the laws of quantum mechanics. In the last two decades, we heard many times that the mysterious gigantic machine was coming, and with no exception that we were left disappointed with only a laboratory setup of a few qubits that can do nothing useful. However, it was totally different this time starting from 2016 when IBM put out a quantum cloud machine with five qubits. It signaled a new race to build a working quantum computer with big companies such as IBM, Google, Microsoft and the like as the competitors. In 2019, Google has demonstrated the solution of a mathematical problem with only 200 seconds in a home-made 53 qubits quantum machine which would have taken 10,000 years for Summit, one of the world’s most powerful supercomputers, to complete, a clear milestone of quantum supremacy. We have now formally entered the Noisy-Intermediate-Scale Quantum (NISQ) era.

    In the NISQ era, quantum machine has already the potential to solve practical problems that would not be possible for the most powerful present-day classical machine. The problem demonstrated by Google is a pure mathematical problem to show the supremacy of quantum computer and has no practical relevance. Therefore, finding quantum algorithms for practical problems that can be solved in NISQ computer is a primary task in quantum computing. As one of the most promising applications of quantum computers in the near future, quantum chemistry simulation is expected most likely to show the advantages at the practical applications in NISQ. Chemistry simulation consumes tremendous computational resources, which makes classical computers impossible to accurately calculate the ground state energy and electronic structure of large molecules. Quantum chemistry simulation is expected to reduce computational complexity exponentially and realizes efficient simulation for these complex molecules that are playing important roles in bio-pharmaceuticals, new materials and energy, high-temperature superconductivity and so on. Designing suitable and efficient quantum algorithms are the key to performing efficient quantum simulation.

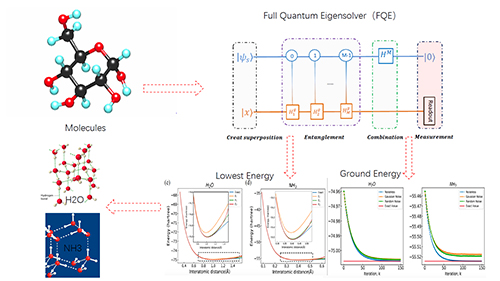
    In a recent paper published in Research [A Full Quantum Eigensolver for Quantum Chemistry Simulations, Research, 2020, 1486935, DOI: 10.34133/2020/1486935],  Shi-Jie Wei, Hang Li and Gui-Lu Long from Beijing Academy of Quantum Information Sciences and Tsinghua University, reported a full quantum eigensolver (FQE) for quantum chemistry simulations. The authors constructed a quantum algorithm based on quantum gradient descent to calculate the ground state energy and state of a molecule. Compared to other quantum eigensolver such as the variational eigensolver (VQE) which works partly in quantum machine and partly in classical machine iteratively, which is attractive for studies in the NISQ computer, FQE removes the classical optimizer and performs all the calculations in full in the quantum machine. FQE can perform the calculations all in the quantum machine because it has used the linear combination of unitaries (LCU) method or the duality quantum computing approach proposed in 2002 by one of the authors [Gui-Lu Long. "General quantum interference principle and duality computer." Comm Theor Phys 45(5) (2006): 825; SPIE conference on “Fluctuations and Noise in Photonics and Quantum Optics” in 2002]. FQE achieves a faster convergence. In FQE, one feeds a molecule into the program and obtains an explicit quantum circuit automatically after some pre-calculations. Then the FQE program calculates the energy of the molecule together with the corresponding electronic structures. FQE can also process the most difficult part of quantum chemistry, finding the most stable structure of the molecules by varying the interatomic distances of a molecule, obtaining the lowest energy in the potential-energy surface, which corresponds to the most stable structure of the molecules.

     Because FQE is fully implemented in a quantum computer, it can not only work in NISQ computer, but also work in future large-scale fault-tolerant quantum computer. With improvement and additions, FQE can be developed into a general-purpose powerful platform for solving quantum chemistry problems for a long time in the future ahead.